Nano From Quantum Perspective

Classical mechanics gives the perspective of trajectories of macroscopic particles. Since the dimension of the nanomaterial lies in nano scale, it cannot be understood without considering quantum mechanics. In quantum mechanics we have to consider particle as well as wave characteristics of a matter. Louis de Brogile first propounded this wave-particle dual nature of material to address the apparent anomaly observed in black body radiation specifically, the ultraviolet catastrophe.

What is quantum mechanics?

• Quantum mechanics is a branch of physics that was developed in the early part of the twentieth century. 
• It is extremely effective in describing the behavior of atoms, molecules, nuclei, and, most recently, nanoparticles.
•  Quantum mechanics is based on the concept that matter has wave properties. 
• Quantum mechanics is used to calculate the energies of particles confined to a finite (and small) region of space. 
• The uncertainty principle is a consequence of quantum mechanics, as is the phenomenon of electron tunneling. 
• Quantum mechanics is about probability.

Planck's Hypothesis

    Planck postulated that the energy of light is proportional to the frequency, and the constant that relates then is known as Planck's contant(h).

        E=h*f

    where, E=Energy

h=Planck's Constant

f=Frequency of the Radiation

His work led to Albert Einstein determining that light exists in discrete quanta of energy or photons.

These principle give rise to 3 postulates: 

1. Light is absorbed or emitted in a little packet or bundle called a quantum
2. Quantum minimum amount of energy that can be lost or gained by an atom.
3. Energies are quantized (think steps, not as ramp)

Density of States(DOS)

The density of states(DOS) is essentially the number of different states at a particular energy level that electrons are allowed to occupy, i.e. the number of the electron states per unit volume per unit energy. The density of states vary according to the dimension of the material.

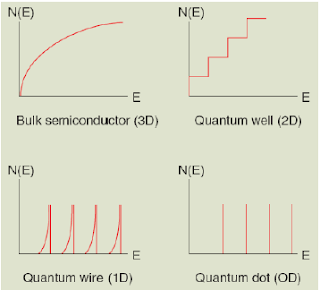DOS of various Dimension material

• DOS for bulk semiconductor is parabolic with respective to the energy level.
• DOS of the quantum well or 2 dimension material or the quantum well is step-wise. It means that for a certain range of energy the number of state which electron can occupy is same unlike bulk material. 
• DOS for 1D material is toothe shape and there is DOS for certain energy level only as we can see in the picture above.
• DOS for 0D material from different from the rest as we can see in the above picture as it is discrete in nature and only certain energy level electron can occupy and thus have DOS. 

Density of State can give us clear picture why nanomaterial is different from bulk material and even why the property of 1D, 2D and 0D material varies.

Particle in a Box

In 1926 Erwin Schrödinger proposed the wave equation that is used to describe how waves of matter (or the wave function) propagate in space and time. The wave function is similar to our identity card and contains all of the information that can be known about a particle The wave function of a free particle moving along the x axis is given by The probability of finding a particle in a position inside the box is given by:

𝚿(𝒙)=𝑨𝐬𝐢𝐧(𝟐𝝅𝒙/𝝀)=𝑨𝐬𝐢𝐧(𝒌𝒙)

The probability of finding a particle in a position inside the box is given by:

                                 

The de Broglie wavelength 𝝀=𝒉/𝒑 which is quantized, and hence the

momentum(p) is also quantized. The kinetic energy (En) associated with the motion of the particle is also quantized and given by:

This is an important result that tells us:

• The energy of a particle is quantized, and
• The lowest possible energy of a particle is NOT zero This is called the     zero point energy and means the particle can never be at rest because it always has some kinetic energy.

This is also consistent with the Heisenberg Uncertainty Principle: if the particle had zero energy, we would know where it was in both space and time.